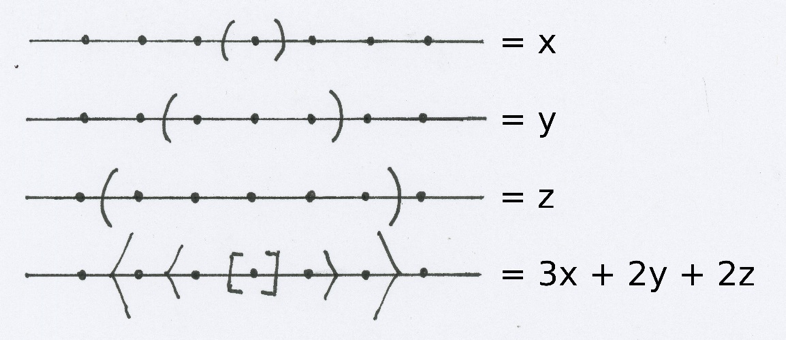Pitch Bracket Notation
The music staff has been a fundamental component of music notation since its introduction, nearly 1000 years ago. Since that time, countless innovations in music notation have been incorporated into the staff, including the key signature, time signature, accidentals, ornamentation, and dynamics. It’s hard to imagine written music without a staff. Yet there is one basic element of music which is not plainly represented on the staff: the interval. The pitch of a note is determined by its vertical position on the staff lines. Consequently, the interval between two notes can only be determined by visually counting the number of lines and spaces between them. Musicians become familiar with the appearance of intervals on the staff and can usually read them fast enough to play by sight. Nevertheless, reading intervals on the staff is cognitively more complicated than, for example, distinguishing a whole note from a quarter note. This is unfortunate because when we listen to music, we care about the intervals between notes, not the absolute position of the notes on the staff. The new music notation presented here illustrates the beauty and symmetry of music written in a language of intervals rather than staff notation.
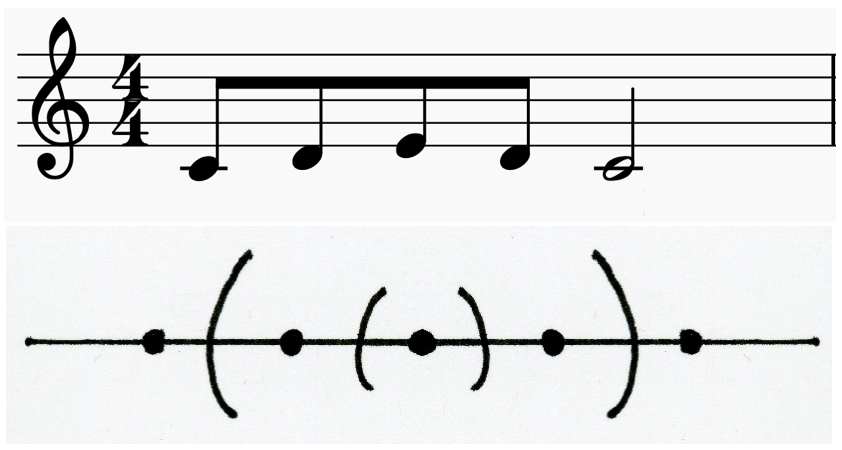
In pitch bracket notation, the staff is replaced with a single horizontal line. The melody line has a particular pitch, like a staff line, but the pitch can be changed by writing pitch brackets. The parenthesis pitch bracket changes the melody line by one scale step. The opening parenthesis adds one scale step while the closing parenthesis subtracts one scale step. The angle bracket changes the melody line by two steps and the square bracket by three steps. A simple C major melody is rendered in pitch bracket notation below. Melody lines always start on the first scale step. The angle brackets raise the melody line by 3rds up to the 5th scale step. Then the square bracket raises the melody line to the octave. The melody line then descends by the same intervals. It is not necessary to write a key signature because pitch is relative to the scale steps, not an absolute key center. It is however necessary to indicate the scale, major or minor, at the start of the melody line. The major scale is assumed unless otherwise noted.

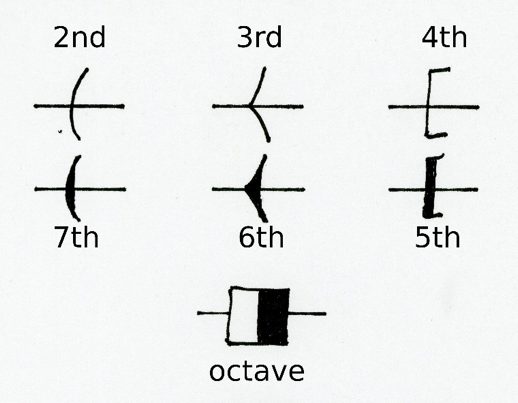
The intervals we find in music, each have distinct qualities and harmonic roles. So why do they look so similar on the staff? In pitch bracket notation each interval has a distinct bracket shape. The figure below shows the pitch brackets along with their corresponding intervals. By combining these brackets in various configurations, any melody can be written. Once written in pitch bracket notation, many interesting harmonic and melodic patterns become plainly visible on the melody line.
An example with real music is now appropriate. The four melody lines below, read left to right, top to bottom, make up the theme of Debussy’s Girl with the Flaxen Hair. In the first two lines, the symmetry of falling and raising 3rds is clearly visible. Third line shows the melody briefly raising a 3rd above the tonic and then falling a 3rd below the tonic. The final line shows the melody stepping down to the subdominant.
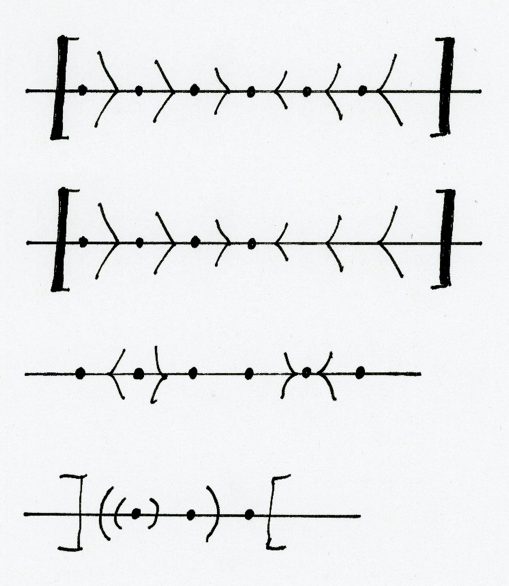
Beethoven’s 5ths symphony begins with those famous four notes which are, in scale degrees, 5 5 5 3. Only Beethoven could have produced such dramatic, thrilling variations on such a trivial motif. The melody lines below show how Beethoven constructed these variations by tweaking the motif’s intervals. Two nested pairs of brackets can be seen. The inside brackets define the interval between the first three notes and the final note. The outside brackets define the interval between the fourth note and the tonic.
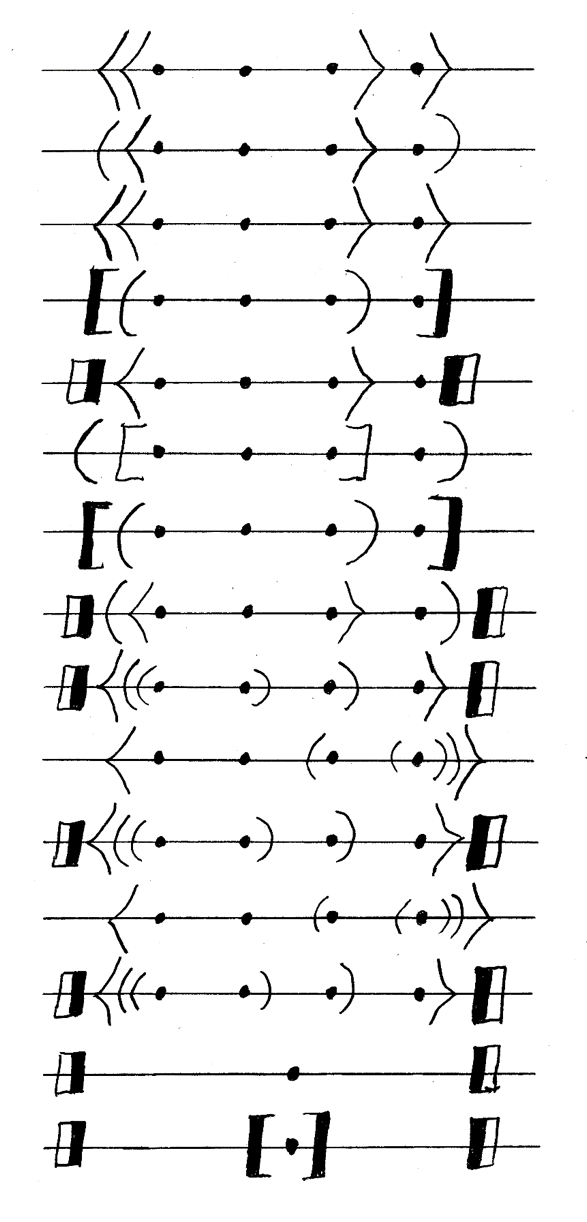
Many additional elements of this notation have been omitted here for the sake of brevity. Timing and rhythm, musical form and many other elements are currently being developed and discussed. One fascinating direction which may interest musicians and mathematicians alike, is the application of algebra to pitch bracket notation. I have shown that some music can be written as algebraic combination of what might be called melodic components. The lines below show a very simple example of pitch bracket algebra. I hope my work on pitch bracket notation encourages people to explore new directions in music notation, performance, and composition. You can find out more about pitch bracket notation at pitchbracket.com.